True Count Blackjack
Running Count / True Count Graph The running count (grey graph line) keeps track of the number of high vs. Low cards using the classic Hi-Lo count strategy, where cards 2-3-4-5-6 have a value of +1, cards 7-8-9 have a value of 0, and cards 10-J-Q-K-A have a value of -1. The count at the beginning is zero. Counting cards in blackjack is tough, and though not illegal, it's frowned upon and can get you booted from casinos. But if you already have a firm grasp of the game, it's a great way to help you.
Blackjack Running Count, True Count, and Player Advantage Simulator
The following Excel spreadsheet simulates the dealing of 6 decks of cards at random. It graphs the running count, true count, and player advantage as the cards are dealt. When counting cards, you should make your minimum waiting bet when the player advantage is zero or negative. When the player advantage goes positive, you should bet a percentage of your bankroll equal to your player advantage, according to the Kelly Criterion. Instructions
- If necessary, bring the two graphs into view using the scroll bar:
Running count / True count
Player advantage % - To deal another 6 decks and update the graphs, first click to select any cell (such as K4), then press the F9 key. Press F9 again for another 6 decks. If your device does not have an F9 key, reload the page.
Notes
- To see graphs for a single-deck or double-deck game, click the 'Single' or '2 decks' tab at the bottom of the window.
- If you accidentally modify a cell value or formula, you might ruin the graph. To fix it, reload the web page.
- Another way to deal 6 decks is to click the Refresh icon( )at the bottom of the spreadsheet window.
Instructional Videos
Running Count | True Count | Two Decks | Six Decks 3:2 6:5 |
Running Count / True Count Graph
The running count (grey graph line) keeps track of the number of high vs. low cards using the classic Hi-Lo count strategy, where cards 2-3-4-5-6 have a value of +1, cards 7-8-9 have a value of 0, and cards 10-J-Q-K-A have a value of -1. The count at the beginning is zero. The count at the end is also zero, because the numbers of low and high cards are balanced.As the cards are dealt, the count randomly varies between positive and negative values, depending on whether more low or high cards are dealt out. The graph is equally likely to be above or below zero.
The true count (blue graph line in top graph) is the running count divided by the number remaining decks to be dealt. This adjusts the running count to determine the true effect, fraction-wise, on the probability of dealing out high or low cards from the remaining decks. For this reason, I prefer to call this the 'true-
 effect count' or 'one-deck equivalent count.'
effect count' or 'one-deck equivalent count.'Player Advantage Graph
The player advantage graph shows your advantage as a percentage of your bet. For example, when your advantage is 1.00 percent and you make a $20 bet, your average win on that bet will be 20 cents. Not a huge advantage by any means! You have an advantage when the graph goes above zero, as indicated by the solid black horizontal line. The dashed horizontal purple line - - - - - - - represents the basic strategy player edge for a typical good 6-deck game, -0.6 percent. The player advantage graph is centered on the dashed purple line; it is equally likely to be above or below this line. Thus, you play at a disadvantage more than half the time.
The player advantage graph is shown solid for the first 4.5 decks and dashed for the remaining 1.5 decks. This is because most casinos shuffle when there are 1.5 decks remaining. That means you will not have any opportunities to make bets in the dashed portions of the graph.
You can place a bet only at the end of each round. At an uncrowded table, maybe 12 or so cards are dealt in each round, on average. Therefore, I've spaced the card number labels at the bottom at intervals of 12. These represent (approximately) the intervals at which you can place bets.
Cell N2 contains the basic strategy player advantage at the start of the game, -0.60 percent by default. For a game that pays only 6:5 on blackjack, the player advantage is worse by 1.4 percent. To see the effect of a 6:5 payoff, enter -2.00 into cell N2.
Player Advantage Pie Chart
The pie chart shows the relative amounts of time that you play at an advantage, at a disadvantage, and even with the casino. A player edge between -0.25 and +0.25 percent is considered even. The pie chart applies only to the part of the graph before the shuffle. For More Information
For more about card counting, see Does Card Counting Really Work? (web page or YouTube video)Or see the whole YouTube playlist 'Easy basic strategy, house edge, and card counting'
For another good introduction to card counting, see The Wizard of Odds website
For a very good and free online book on blackjack, see ModernBlackjack, An Illustrated Guide to Blackjack Advantage Play, by Norman Wattenberger
For a detailed technical look at the game, see Wattenberger's How Blackjack Works
Card counting is one of those rare gambling strategies that can actually work. Most so-called gambling strategies are just systems relying on the gambler’s fallacy, and in the long run, they fail.
But counting cards actually gives a demonstrable, mathematical edge over the casino.
And the casinos know it.
As a result, they’ve instituted countermeasures to make it harder to get an edge. One of these countermeasures is the use of multiple decks. It’s harder to get an edge when facing eight decks in a shoe.
And the use of those additional decks requires you to convert the running count to a true count. This post focuses on how and why you need to convert the running count to a true count.
But first, I’ll explain some of the basics of card counting.
The process is deceptively simple, but it takes practice and discipline to make any money with it. For purposes of introducing these concepts, I’m going to use the “Hi Lo Count,” which is the most widely used card counting system. It’s also one of the easiest to understand.
If you’ve ever read an actual book about blackjack or counting cards, you’ve probably seen some reference to it. Harvey Dubner first introduced this system in 1963, but it’s as effective now as it was 50 years ago.
Stanford Wong covers the Hi-Lo System in his book Professional Blackjack, and Don Schlesinger goes into more detail about the system in Blackjack Attack. Beat the Dealer by Ed Thorp features a card counting system that’s almost the same as the Hi-Lo System, too.
Being able to convert the running count to the true count is a skill necessary for any counter using any system — but only if they’re playing in a game with multiple decks.
How and Why Counting Cards Works
Most gambling systems involve raising and lowering the size of your bets based on what’s happened on previous outcomes. Most of them don’t work.
Here’s why.
Almost all games feature independent, random trials. The probabilities don’t change based on what happened during the previous trial.
For example, you roll a pair of dice. The probabilities are based on the number of sides each die has.
Once you’ve rolled the dice, and you’re ready to roll them again, the dice still have the same number of sides.
The formula for an event’s probability is the number of ways that event can happen divided by the total number of possible events.
When rolling dice, for example, you have 36 possible outcomes. Only one of those outcomes totals 2, so the probability of rolling a 2 on a pair of dice is 1/36.
If you roll a 2, then roll the dice again, the probability of rolling a 2 on the next roll is still 1/36. It doesn’t go up or down based on what happened on the last roll.
But in blackjack, the probabilities change as the cards are dealt because the number of possible outcomes changes.
- You’re playing blackjack from a single deck. The probability of a card being an ace is 4/52, or 1/13.
- You deal out two hands, and each of those hands has an ace.
- The probability of getting an ace on the next card has changed.
- For one thing, now there are only 48 possible outcomes — that’s how many cards are left in the deck.
- There are also only two aces left in the deck, so the probability of getting an ace drops to 2/48, or 1/24.
There’s a big difference between a 12 to 1 shot at an ace and a 23 to 1 shot at an ace.
Why the Aces and Tens Are Important
I used aces in the previous example, but I could have also used 10s. There are 16 cards worth 10 points in a blackjack deck — four each of 10s, jacks, queens, and kings. The probability of being dealt a card worth 10 is 16/52, or 4/13.
The 10s are as important as the aces, but you’re more likely to get a 10.
Here’s why that’s important.
When you win at blackjack, you get paid off at even money most of the time. The exception is when you have a two-card hand that totals 21.
That’s called a “blackjack” or a “natural,” and that pays off at 3 to 2.
Since the decks are shuffled and randomized, the aces and 10s might be disproportional based on where they fell in the deck. If a lot of the aces and 10s have already been dealt, your probability of getting a blackjack decreases.
But if you have a higher-than-usual proportion of aces and 10s in the deck, your probability of getting a blackjack increases.
If you bet more when you have a better probability of getting a 3 to 2 payout, you’ll wind up with a mathematical edge over the casino.
And counting cards enables you to track that ratio.
How and Why to Use the Hi-Lo Count as a Beginner
The Hi-Low Count, or the High-Low Count, assigns a value of +1 to the lower-valued cards in the deck and a value of -1 to the higher-valued cards in the deck. Mid-sized cards have a value of 0.
As you see these cards get played, you adjust the count according to which cards have already been dealt.
- When you see a 2, 3, 4, 5, or 6, you add 1 to the count.
- When you see a 10 or an ace, you subtract 1 from the count.
- For counting purposes, you ignore the 7, 8, and 9.
This gives you an idea of your edge at any given time, until the casino dealer shuffles the cards back in the deck.
When the count is positive, you have a proportionally higher number of favorable cards in the deck.
When the count is 0 or negative, you have a proportionally lower number of favorable cards in the deck.
You raise the size of your bets when the count is positive.
You bet your minimum when the count is 0 or negative.

It’s almost just that simple, too.
Basics Of Card Counting
You’ll find different guidelines for how much to bet based on the count, but the easiest system I learned was to start with a single unit — your minimum. Then decide what your betting spread will be, keeping in mind that the bigger the spread is, the more aggressive you’re being.
The more aggressive you are, the more likely you are to catch heat from the casino.
Then you add the count to 1 to decide the size of your bet.
- You’re playing at a table with a minimum bet of $5.
- You decide you’re going to use that as your base betting unit, and you’re going to have a betting spread of 1 to 4 units, based on the count.
- You’ll be betting in amounts of $5, $10, $15, or $20 — 1 unit, 2 units, 3 units, or 4 units, respectively.
- When the count is 0 or less, you’ll bet $5.
- When the count is +1, you’ll bet $10.
- When the count is +2, you’ll bet $15.
- When the count is +3 or higher, you’ll bet $20.
This works fine as long as you’re playing in a game being dealt from a single deck.
But if you’re playing in a game being dealt from multiple decks in a shoe, you need to know how to convert the running count to the true count.
Converting the Running Count to a True Count
Converting the running count to a true count isn’t as hard as many people think. It’s just a matter of estimating how many decks are left in the shoe. You divide the running count by the number of decks left to get the true count.
Most people get hung up on being too accurate. All you need is an estimate. Rounding off is your friend here.
Take a look at the example below.
The running count is +9, and you estimate that there are four decks left in the show.
9 divided by 4 is 2.25, but you can just round that off to 2 and make your decisions based on a true count of +2.
You’ll find card counting systems which eliminate the need to convert to a true count, but I don’t think they’re necessary. If you’re able to keep up with the running count accurately, you’re also smart enough to convert that to a true count.
Another advantage to doing the true count conversion is that it reminds you to start over at 0 when the dealer reshuffles the cards. One time, I was drinking while I was counting cards, and I was also chatting with my wife while we were playing.
I bet according to the count, even though the dealer had already shuffled.
Your edge is too small when counting cards to let small mistakes like this slip into your game.
The counting systems that eliminate the need to convert into a true count are usually unbalanced systems. They have a different number of positive and negative values, so if you counted through a single deck, you’d wind up with something other than 0.
With these other counting systems, you’ll also often start your count with a number other than 0. This is to compensate for the number of decks in play.
In these unbalanced systems, you have to jump through so many hoops that you’re better off just doing the true count conversion.
- With the Hi-Lo System, a single deck of cards has 24 cards worth +1 and 24 cards worth -1.
- They even out.
- But with an unbalanced system, you might have 24 cards worth +1 and 26 cards worth -1.
Count through a deck with this system, and you’ll end up with -2 instead of 0.
Why the Number of Decks Matters
True Count Vs Running Count Blackjack
When I first learned about card counting, I couldn’t figure out why the number of decks would matter. After all, the ratio of aces and 10s to the other cards is the same regardless of how many decks there are, right?
This is true before you deal any cards, yes. With eight decks, you have 32 aces and 516 totals cards, which is still 1 in 13.
But the ratios change as the cards are dealt, and with more cards in the deck, the ratios are different.
- You’re playing in a single-deck game, and all four aces have already been played.
- Your probability of getting an ace is now 0.
- Later, you’re playing in an eight-deck game, and you see four aces come out.
- Your probability of getting an ace isn’t 0, because there are still 12 aces left in the deck. The probability isn’t as good, but it’s not 0, either.
- That’s why the number of decks matters.
- The numbers you’re using to divide by when determining the ratio are bigger.
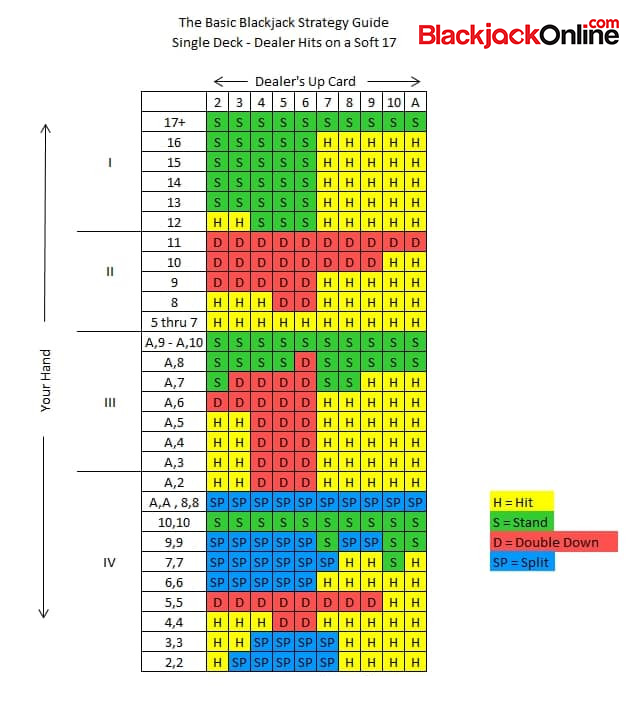
Changing Your Basic Strategy Based on the True Count
Changing the size of your bets isn’t the only way to get an edge when playing blackjack. Skilled counters also know when to deviate from basic strategy.
In blackjack, there’s a correct mathematical play in every situation. That’s the play with the best expected return. In some situations, the expected return is negative no matter which choice you make. In those situations, you want the expected return that’s the least negative.
The first and easiest basic strategy deviation for the card counter is insurance. Basic strategy says you should never take insurance. After all, it’s a sucker bet.
But when the true count is +3 or greater, insurance becomes a positive expectation bet.
That’s because as the ratio of high cards changes, the dealer has a better probability of getting a blackjack, too. When that probability gets high enough, insurance becomes a positive expectation bet.
When you have a hard 16 versus a dealer’s 10, you also have a strategy change to consider. The correct strategy play is to hit because of the likelihood that the dealer has a high total. You’re still likely to bust, but it’s still the correct play.
But if you’re counting cards and the count is positive, you should stand instead of hitting. Even a slightly higher positive count results in a higher probability of busting with that extra card.
When you have a hard 15 versus a dealer 10, you also have a basic strategy adjustment to make, but you only make the adjustment if the true count is +4 or higher. This is another hand where you would normally hit if you’re following basic strategy.
But if the count is +4 or higher, you’ll stand instead.
If you’re following basic strategy, you also know that you never split 10s.
Card Counting
But if the dealer is showing a 5, and if the true count is +5 or more, the correct play is to split those 10s. That’s because you have such a high percentage of getting an ace or a 10 as your next card that it’s worth your while to get two really strong hands into play against the dealer.
After all, the dealer has a lousy hand with that 5 showing.
A pair of 10s versus a dealer 6 is the same, but the true count only has to be +4 to split instead of +5.
If you have a hard 10 when the dealer is showing a 10, you’ll deviate from basic strategy if the true count is +4. Normally, in that situation, you’d hit, but if the count is this positive, you’ll double down instead.
If you have a hard 12 versus a dealer 3, you’ll deviate from basic strategy if the true count is +2 or higher. Ordinarily, you’d hit this hand, but if the count is this positive, you’ll stand, hoping that the dealer will bust when she gets a 10.
A 12 versus a dealer 2 is the same thing, but the true count needs to be +3 or higher before you deviate from basic strategy.
If you have an 11 versus a dealer’s ace, you’ll deviate from basic strategy when the count is +1 or better. Normally, you’d hit this hand, but when the count is positive, you’ll double down.
If you have a 9 versus a dealer 2, you’d usually hit, but if the count is +1 or higher, you’ll double down.
Those are the ten most important basic strategy deviations based on the true count. You can find more basic strategy deviations by searching for phrases like “Illustrious 18” and “Fab 4.” (The “Fab 4” describes when you should surrender based on the count.
Conclusion
Counting cards can be a lot of fun and can get you an edge over the casino.
But if you’re playing in a game with multiple decks, you’ll need to know how to get the true count.
Luckily, it’s easy to do.
You just divide the running count by the number of decks left in the shoe.
And don’t forget, an estimate is all you need. You should just round off.
Please enable JavaScript to view the comments powered by Disqus.